Embark on a mathematical odyssey with our series and sequences practice problems, meticulously crafted to unravel the intricacies of summation and unlock your problem-solving prowess.
Delve into the fundamental concepts of arithmetic and geometric progressions, equipping yourself with the essential formulas for calculating their sums. Practice makes perfect, and our curated table of problems provides a structured path from beginner-friendly challenges to mind-boggling conundrums.
Series and Sequences Practice Problems

Series and sequences are fundamental concepts in mathematics that involve studying the patterns and relationships between numbers. This article provides an overview of series and sequences, practice problems, and their applications in real-world scenarios.
Series and Sequences Concepts
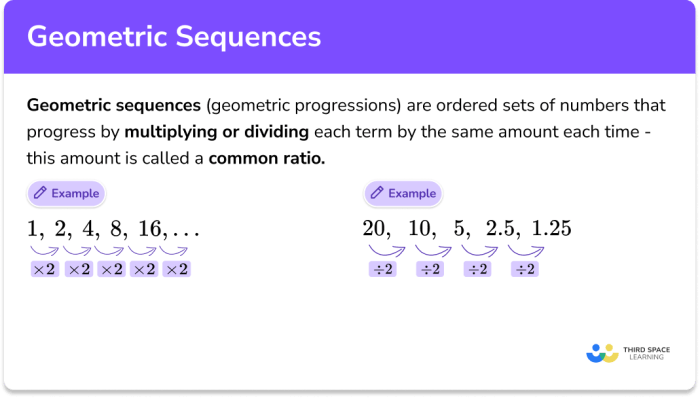
A series is a sum of terms, while a sequence is an ordered list of terms. Arithmetic series and sequences involve a constant difference between terms, while geometric series and sequences involve a constant ratio between terms.
The sum of an arithmetic series with n terms, first term a, and common difference d is given by the formula: Sn= n/2 – (2a + (n-1)d)
The sum of a geometric series with n terms, first term a, and common ratio r is given by the formula: Sn= a(1 – r n) / (1 – r)
Solving Practice Problems
| Problem Number | Problem Statement | Solution | Difficulty Level |
|---|---|---|---|
| 1 | Find the sum of the first 10 terms of the arithmetic series 2, 5, 8, … | S10 = 10/2
|
Easy |
| 2 | Find the sum of the first 5 terms of the geometric series 1, 2, 4, … | S5= 1(1
|
Medium |
Methods for Solving Problems, Series and sequences practice problems
Solving series and sequences problems involves identifying the type of series or sequence, using the appropriate formula, and applying algebraic techniques. For arithmetic series, finding the common difference is crucial, while for geometric series, finding the common ratio is essential.
A flowchart can be used to illustrate the problem-solving process, including steps for identifying the series type, calculating the common difference or ratio, and applying the appropriate formula.
Applications in Real-World Scenarios
| Application Area | Problem Type | Solution Approach |
|---|---|---|
| Finance | Compound interest | Use geometric series to calculate the future value of an investment. |
| Physics | Projectile motion | Use arithmetic series to calculate the distance traveled by an object in equal time intervals. |
Popular Questions
What is the difference between an arithmetic and geometric sequence?
An arithmetic sequence has a constant difference between consecutive terms, while a geometric sequence has a constant ratio between consecutive terms.
How do I find the sum of an arithmetic series?
Use the formula Sn = n/2 – (a1 + an), where Sn is the sum of the first n terms, a1 is the first term, and an is the nth term.
What is the formula for the sum of a geometric series?
Use the formula Sn = a1 – (1 – r^n) / (1 – r), where Sn is the sum of the first n terms, a1 is the first term, and r is the common ratio.